Uniform 7-polytope

Graphs of three regular and related uniform polytopes

7-simplex				Rectified 7-simplex				Truncated 7-simplex
Cantellated 7-simplex				Runcinated 7-simplex				Stericated 7-simplex
Pentellated 7-simplex						Hexicated 7-simplex
7-orthoplex				Truncated 7-orthoplex				Rectified 7-orthoplex
Cantellated 7-orthoplex				Runcinated 7-orthoplex				Stericated 7-orthoplex
Pentellated 7-orthoplex				Hexicated 7-cube				Pentellated 7-cube
Stericated 7-cube				Cantellated 7-cube				Runcinated 7-cube
7-cube				Truncated 7-cube				Rectified 7-cube
7-demicube				Cantic 7-cube				Runcic 7-cube
Steric 7-cube				Pentic 7-cube				Hexic 7-cube
321				231				132

In seven-dimensional geometry, a 7-polytope is a polytope contained by 6-polytope facets. Each 5-polytope ridge being shared by exactly two 6-polytope facets.

A uniform 7-polytope is one whose symmetry group is transitive on vertices and whose facets are uniform 6-polytopes.

Regular 7-polytopes are represented by the Schläfli symbol {p,q,r,s,t,u} with u {p,q,r,s,t} 6-polytopes facets around each 4-face.

There are exactly three such convex regular 7-polytopes:

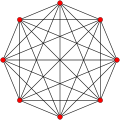
1. {3,3,3,3,3,3} - 7-simplex
2. {4,3,3,3,3,3} - 7-cube
3. {3,3,3,3,3,4} - 7-orthoplex

There are no nonconvex regular 7-polytopes.

The topology of any given 7-polytope is defined by its Betti numbers and torsion coefficients.^[1]

The value of the Euler characteristic used to characterise polyhedra does not generalize usefully to higher dimensions, whatever their underlying topology. This inadequacy of the Euler characteristic to reliably distinguish between different topologies in higher dimensions led to the discovery of the more sophisticated Betti numbers.^[1]

Similarly, the notion of orientability of a polyhedron is insufficient to characterise the surface twistings of toroidal polytopes, and this led to the use of torsion coefficients.^[1]

Uniform 7-polytopes with reflective symmetry can be generated by these four Coxeter groups, represented by permutations of rings of the Coxeter-Dynkin diagrams:

#	Coxeter group			Regular and semiregular forms	Uniform count
1	A7	[36]		7-simplex - {36},	71
2	B7	[4,35]		7-cube - {4,35}, 7-orthoplex - {35,4}, 7-demicube - h{4,35},	127 + 32
3	D7	[33,1,1]		7-demicube, {3,34,1}, 7-orthoplex, {34,31,1},	95 (0 unique)
4	E7	[33,2,1]		321 - 132 - 231 -	127

Prismatic finite Coxeter groups
#	Coxeter group		Coxeter diagram
6+1
1	A6A1	[35]×[ ]
2	BC6A1	[4,34]×[ ]
3	D6A1	[33,1,1]×[ ]
4	E6A1	[32,2,1]×[ ]
5+2
1	A5I2(p)	[3,3,3]×[p]
2	BC5I2(p)	[4,3,3]×[p]
3	D5I2(p)	[32,1,1]×[p]
5+1+1
1	A5A12	[3,3,3]×[ ]2
2	BC5A12	[4,3,3]×[ ]2
3	D5A12	[32,1,1]×[ ]2
4+3
1	A4A3	[3,3,3]×[3,3]
2	A4B3	[3,3,3]×[4,3]
3	A4H3	[3,3,3]×[5,3]
4	BC4A3	[4,3,3]×[3,3]
5	BC4B3	[4,3,3]×[4,3]
6	BC4H3	[4,3,3]×[5,3]
7	H4A3	[5,3,3]×[3,3]
8	H4B3	[5,3,3]×[4,3]
9	H4H3	[5,3,3]×[5,3]
10	F4A3	[3,4,3]×[3,3]
11	F4B3	[3,4,3]×[4,3]
12	F4H3	[3,4,3]×[5,3]
13	D4A3	[31,1,1]×[3,3]
14	D4B3	[31,1,1]×[4,3]
15	D4H3	[31,1,1]×[5,3]
4+2+1
1	A4I2(p)A1	[3,3,3]×[p]×[ ]
2	BC4I2(p)A1	[4,3,3]×[p]×[ ]
3	F4I2(p)A1	[3,4,3]×[p]×[ ]
4	H4I2(p)A1	[5,3,3]×[p]×[ ]
5	D4I2(p)A1	[31,1,1]×[p]×[ ]
4+1+1+1
1	A4A13	[3,3,3]×[ ]3
2	BC4A13	[4,3,3]×[ ]3
3	F4A13	[3,4,3]×[ ]3
4	H4A13	[5,3,3]×[ ]3
5	D4A13	[31,1,1]×[ ]3
3+3+1
1	A3A3A1	[3,3]×[3,3]×[ ]
2	A3B3A1	[3,3]×[4,3]×[ ]
3	A3H3A1	[3,3]×[5,3]×[ ]
4	BC3B3A1	[4,3]×[4,3]×[ ]
5	BC3H3A1	[4,3]×[5,3]×[ ]
6	H3A3A1	[5,3]×[5,3]×[ ]
3+2+2
1	A3I2(p)I2(q)	[3,3]×[p]×[q]
2	BC3I2(p)I2(q)	[4,3]×[p]×[q]
3	H3I2(p)I2(q)	[5,3]×[p]×[q]
3+2+1+1
1	A3I2(p)A12	[3,3]×[p]×[ ]2
2	BC3I2(p)A12	[4,3]×[p]×[ ]2
3	H3I2(p)A12	[5,3]×[p]×[ ]2
3+1+1+1+1
1	A3A14	[3,3]×[ ]4
2	BC3A14	[4,3]×[ ]4
3	H3A14	[5,3]×[ ]4
2+2+2+1
1	I2(p)I2(q)I2(r)A1	[p]×[q]×[r]×[ ]
2+2+1+1+1
1	I2(p)I2(q)A13	[p]×[q]×[ ]3
2+1+1+1+1+1
1	I2(p)A15	[p]×[ ]5
1+1+1+1+1+1+1
1	A17	[ ]7

The A₇ family has symmetry of order 40320 (8 factorial).

There are 71 (64+8-1) forms based on all permutations of the Coxeter-Dynkin diagrams with one or more rings. All 71 are enumerated below. Norman Johnson's truncation names are given. Bowers names and acronym are also given for cross-referencing.

See also a list of A7 polytopes for symmetric Coxeter plane graphs of these polytopes.

A7 uniform polytopes
#	Coxeter-Dynkin diagram	Truncation indices	Johnson name Bowers name (and acronym)	Basepoint	Element counts
					6	5	4	3	2	1	0
1		t0	7-simplex (oca)	(0,0,0,0,0,0,0,1)	8	28	56	70	56	28	8
2		t1	Rectified 7-simplex (roc)	(0,0,0,0,0,0,1,1)	16	84	224	350	336	168	28
3		t2	Birectified 7-simplex (broc)	(0,0,0,0,0,1,1,1)	16	112	392	770	840	420	56
4		t3	Trirectified 7-simplex (he)	(0,0,0,0,1,1,1,1)	16	112	448	980	1120	560	70
5		t0,1	Truncated 7-simplex (toc)	(0,0,0,0,0,0,1,2)	16	84	224	350	336	196	56
6		t0,2	Cantellated 7-simplex (saro)	(0,0,0,0,0,1,1,2)	44	308	980	1750	1876	1008	168
7		t1,2	Bitruncated 7-simplex (bittoc)	(0,0,0,0,0,1,2,2)						588	168
8		t0,3	Runcinated 7-simplex (spo)	(0,0,0,0,1,1,1,2)	100	756	2548	4830	4760	2100	280
9		t1,3	Bicantellated 7-simplex (sabro)	(0,0,0,0,1,1,2,2)						2520	420
10		t2,3	Tritruncated 7-simplex (tattoc)	(0,0,0,0,1,2,2,2)						980	280
11		t0,4	Stericated 7-simplex (sco)	(0,0,0,1,1,1,1,2)						2240	280
12		t1,4	Biruncinated 7-simplex (sibpo)	(0,0,0,1,1,1,2,2)						4200	560
13		t2,4	Tricantellated 7-simplex (stiroh)	(0,0,0,1,1,2,2,2)						3360	560
14		t0,5	Pentellated 7-simplex (seto)	(0,0,1,1,1,1,1,2)						1260	168
15		t1,5	Bistericated 7-simplex (sabach)	(0,0,1,1,1,1,2,2)						3360	420
16		t0,6	Hexicated 7-simplex (suph)	(0,1,1,1,1,1,1,2)						336	56
17		t0,1,2	Cantitruncated 7-simplex (garo)	(0,0,0,0,0,1,2,3)						1176	336
18		t0,1,3	Runcitruncated 7-simplex (patto)	(0,0,0,0,1,1,2,3)						4620	840
19		t0,2,3	Runcicantellated 7-simplex (paro)	(0,0,0,0,1,2,2,3)						3360	840
20		t1,2,3	Bicantitruncated 7-simplex (gabro)	(0,0,0,0,1,2,3,3)						2940	840
21		t0,1,4	Steritruncated 7-simplex (cato)	(0,0,0,1,1,1,2,3)						7280	1120
22		t0,2,4	Stericantellated 7-simplex (caro)	(0,0,0,1,1,2,2,3)						10080	1680
23		t1,2,4	Biruncitruncated 7-simplex (bipto)	(0,0,0,1,1,2,3,3)						8400	1680
24		t0,3,4	Steriruncinated 7-simplex (cepo)	(0,0,0,1,2,2,2,3)						5040	1120
25		t1,3,4	Biruncicantellated 7-simplex (bipro)	(0,0,0,1,2,2,3,3)						7560	1680
26		t2,3,4	Tricantitruncated 7-simplex (gatroh)	(0,0,0,1,2,3,3,3)						3920	1120
27		t0,1,5	Pentitruncated 7-simplex (teto)	(0,0,1,1,1,1,2,3)						5460	840
28		t0,2,5	Penticantellated 7-simplex (tero)	(0,0,1,1,1,2,2,3)						11760	1680
29		t1,2,5	Bisteritruncated 7-simplex (bacto)	(0,0,1,1,1,2,3,3)						9240	1680
30		t0,3,5	Pentiruncinated 7-simplex (tepo)	(0,0,1,1,2,2,2,3)						10920	1680
31		t1,3,5	Bistericantellated 7-simplex (bacroh)	(0,0,1,1,2,2,3,3)						15120	2520
32		t0,4,5	Pentistericated 7-simplex (teco)	(0,0,1,2,2,2,2,3)						4200	840
33		t0,1,6	Hexitruncated 7-simplex (puto)	(0,1,1,1,1,1,2,3)						1848	336
34		t0,2,6	Hexicantellated 7-simplex (puro)	(0,1,1,1,1,2,2,3)						5880	840
35		t0,3,6	Hexiruncinated 7-simplex (puph)	(0,1,1,1,2,2,2,3)						8400	1120
36		t0,1,2,3	Runcicantitruncated 7-simplex (gapo)	(0,0,0,0,1,2,3,4)						5880	1680
37		t0,1,2,4	Stericantitruncated 7-simplex (cagro)	(0,0,0,1,1,2,3,4)						16800	3360
38		t0,1,3,4	Steriruncitruncated 7-simplex (capto)	(0,0,0,1,2,2,3,4)						13440	3360
39		t0,2,3,4	Steriruncicantellated 7-simplex (capro)	(0,0,0,1,2,3,3,4)						13440	3360
40		t1,2,3,4	Biruncicantitruncated 7-simplex (gibpo)	(0,0,0,1,2,3,4,4)						11760	3360
41		t0,1,2,5	Penticantitruncated 7-simplex (tegro)	(0,0,1,1,1,2,3,4)						18480	3360
42		t0,1,3,5	Pentiruncitruncated 7-simplex (tapto)	(0,0,1,1,2,2,3,4)						27720	5040
43		t0,2,3,5	Pentiruncicantellated 7-simplex (tapro)	(0,0,1,1,2,3,3,4)						25200	5040
44		t1,2,3,5	Bistericantitruncated 7-simplex (bacogro)	(0,0,1,1,2,3,4,4)						22680	5040
45		t0,1,4,5	Pentisteritruncated 7-simplex (tecto)	(0,0,1,2,2,2,3,4)						15120	3360
46		t0,2,4,5	Pentistericantellated 7-simplex (tecro)	(0,0,1,2,2,3,3,4)						25200	5040
47		t1,2,4,5	Bisteriruncitruncated 7-simplex (bicpath)	(0,0,1,2,2,3,4,4)						20160	5040
48		t0,3,4,5	Pentisteriruncinated 7-simplex (tacpo)	(0,0,1,2,3,3,3,4)						15120	3360
49		t0,1,2,6	Hexicantitruncated 7-simplex (pugro)	(0,1,1,1,1,2,3,4)						8400	1680
50		t0,1,3,6	Hexiruncitruncated 7-simplex (pugato)	(0,1,1,1,2,2,3,4)						20160	3360
51		t0,2,3,6	Hexiruncicantellated 7-simplex (pugro)	(0,1,1,1,2,3,3,4)						16800	3360
52		t0,1,4,6	Hexisteritruncated 7-simplex (pucto)	(0,1,1,2,2,2,3,4)						20160	3360
53		t0,2,4,6	Hexistericantellated 7-simplex (pucroh)	(0,1,1,2,2,3,3,4)						30240	5040
54		t0,1,5,6	Hexipentitruncated 7-simplex (putath)	(0,1,2,2,2,2,3,4)						8400	1680
55		t0,1,2,3,4	Steriruncicantitruncated 7-simplex (gecco)	(0,0,0,1,2,3,4,5)						23520	6720
56		t0,1,2,3,5	Pentiruncicantitruncated 7-simplex (tegapo)	(0,0,1,1,2,3,4,5)						45360	10080
57		t0,1,2,4,5	Pentistericantitruncated 7-simplex (tecagro)	(0,0,1,2,2,3,4,5)						40320	10080
58		t0,1,3,4,5	Pentisteriruncitruncated 7-simplex (tacpeto)	(0,0,1,2,3,3,4,5)						40320	10080
59		t0,2,3,4,5	Pentisteriruncicantellated 7-simplex (tacpro)	(0,0,1,2,3,4,4,5)						40320	10080
60		t1,2,3,4,5	Bisteriruncicantitruncated 7-simplex (gabach)	(0,0,1,2,3,4,5,5)						35280	10080
61		t0,1,2,3,6	Hexiruncicantitruncated 7-simplex (pugopo)	(0,1,1,1,2,3,4,5)						30240	6720
62		t0,1,2,4,6	Hexistericantitruncated 7-simplex (pucagro)	(0,1,1,2,2,3,4,5)						50400	10080
63		t0,1,3,4,6	Hexisteriruncitruncated 7-simplex (pucpato)	(0,1,1,2,3,3,4,5)						45360	10080
64		t0,2,3,4,6	Hexisteriruncicantellated 7-simplex (pucproh)	(0,1,1,2,3,4,4,5)						45360	10080
65		t0,1,2,5,6	Hexipenticantitruncated 7-simplex (putagro)	(0,1,2,2,2,3,4,5)						30240	6720
66		t0,1,3,5,6	Hexipentiruncitruncated 7-simplex (putpath)	(0,1,2,2,3,3,4,5)						50400	10080
67		t0,1,2,3,4,5	Pentisteriruncicantitruncated 7-simplex (geto)	(0,0,1,2,3,4,5,6)						70560	20160
68		t0,1,2,3,4,6	Hexisteriruncicantitruncated 7-simplex (pugaco)	(0,1,1,2,3,4,5,6)						80640	20160
69		t0,1,2,3,5,6	Hexipentiruncicantitruncated 7-simplex (putgapo)	(0,1,2,2,3,4,5,6)						80640	20160
70		t0,1,2,4,5,6	Hexipentistericantitruncated 7-simplex (putcagroh)	(0,1,2,3,3,4,5,6)						80640	20160
71		t0,1,2,3,4,5,6	Omnitruncated 7-simplex (guph)	(0,1,2,3,4,5,6,7)						141120	40320

The B₇ family has symmetry of order 645120 (7 factorial x 2⁷).

There are 127 forms based on all permutations of the Coxeter-Dynkin diagrams with one or more rings. Johnson and Bowers names.

See also a list of B7 polytopes for symmetric Coxeter plane graphs of these polytopes.

B7 uniform polytopes
#	Coxeter-Dynkin diagram t-notation	Name (BSA)	Base point	Element counts
				6	5	4	3	2	1	0
1	t0{3,3,3,3,3,4}	7-orthoplex (zee)	(0,0,0,0,0,0,1)√2	128	448	672	560	280	84	14
2	t1{3,3,3,3,3,4}	Rectified 7-orthoplex (rez)	(0,0,0,0,0,1,1)√2	142	1344	3360	3920	2520	840	84
3	t2{3,3,3,3,3,4}	Birectified 7-orthoplex (barz)	(0,0,0,0,1,1,1)√2	142	1428	6048	10640	8960	3360	280
4	t3{4,3,3,3,3,3}	Trirectified 7-cube (sez)	(0,0,0,1,1,1,1)√2	142	1428	6328	14560	15680	6720	560
5	t2{4,3,3,3,3,3}	Birectified 7-cube (bersa)	(0,0,1,1,1,1,1)√2	142	1428	5656	11760	13440	6720	672
6	t1{4,3,3,3,3,3}	Rectified 7-cube (rasa)	(0,1,1,1,1,1,1)√2	142	980	2968	5040	5152	2688	448
7	t0{4,3,3,3,3,3}	7-cube (hept)	(0,0,0,0,0,0,0)√2 + (1,1,1,1,1,1,1)	14	84	280	560	672	448	128
8	t0,1{3,3,3,3,3,4}	Truncated 7-orthoplex (Taz)	(0,0,0,0,0,1,2)√2	142	1344	3360	4760	2520	924	168
9	t0,2{3,3,3,3,3,4}	Cantellated 7-orthoplex (Sarz)	(0,0,0,0,1,1,2)√2	226	4200	15456 × × €4.95 / month Favourites List Audio Listining Color Mode Monthly New Updates Fullname * Email * Credit or debit card Subscribe A PHP Error was encountered Severity: Notice Message: Undefined variable: userid Filename: user/popup_modal.php Line Number: 68 Backtrace: File: /var/www/wikizero/data/www/wikizero.com/application/views/user/popup_modal.php Line: 68 Function: _error_handler File: /var/www/wikizero/data/www/wikizero.com/application/views/page/index.php Line: 478 Function: view File: /var/www/wikizero/data/www/wikizero.com/application/controllers/Main.php Line: 107 Function: view File: /var/www/wikizero/data/www/wikizero.com/index.php Line: 315 Function: require_once "> Subscribe Now Premium Membership × €4.95 € 9.90 / month Create a Premium Account quick and easy Save your Favourite Pages Listen to any page in Audio Colour Night Mode Create your Account Modal title × Close Save changes Cancel Subscription × Uniform_7-polytope" id="cancelSubscription" name="cancelSubscription" class="mb-0"> Are you sure you want to cancel your membership with us? A PHP Error was encountered Severity: Notice Message: Undefined variable: user_membership Filename: user/popup_modal.php Line Number: 192 Backtrace: File: /var/www/wikizero/data/www/wikizero.com/application/views/user/popup_modal.php Line: 192 Function: _error_handler File: /var/www/wikizero/data/www/wikizero.com/application/views/page/index.php Line: 478 Function: view File: /var/www/wikizero/data/www/wikizero.com/application/controllers/Main.php Line: 107 Function: view File: /var/www/wikizero/data/www/wikizero.com/index.php Line: 315 Function: require_once A PHP Error was encountered Severity: Warning Message: Invalid argument supplied for foreach() Filename: user/popup_modal.php Line Number: 192 Backtrace: File: /var/www/wikizero/data/www/wikizero.com/application/views/user/popup_modal.php Line: 192 Function: _error_handler File: /var/www/wikizero/data/www/wikizero.com/application/views/page/index.php Line: 478 Function: view File: /var/www/wikizero/data/www/wikizero.com/application/controllers/Main.php Line: 107 Function: view File: /var/www/wikizero/data/www/wikizero.com/index.php Line: 315 Function: require_once A PHP Error was encountered Severity: Notice Message: Undefined variable: userid Filename: user/popup_modal.php Line Number: 208 Backtrace: File: /var/www/wikizero/data/www/wikizero.com/application/views/user/popup_modal.php Line: 208 Function: _error_handler File: /var/www/wikizero/data/www/wikizero.com/application/views/page/index.php Line: 478 Function: view File: /var/www/wikizero/data/www/wikizero.com/application/controllers/Main.php Line: 107 Function: view File: /var/www/wikizero/data/www/wikizero.com/index.php Line: 315 Function: require_once "> Yes Cancel Continue Subscription Harry Potter Books × A PHP Error was encountered Severity: Notice Message: Undefined variable: products Filename: user/popup_harry_book.php Line Number: 24 Backtrace: File: /var/www/wikizero/data/www/wikizero.com/application/views/user/popup_harry_book.php Line: 24 Function: _error_handler File: /var/www/wikizero/data/www/wikizero.com/application/views/page/index.php Line: 479 Function: view File: /var/www/wikizero/data/www/wikizero.com/application/controllers/Main.php Line: 107 Function: view File: /var/www/wikizero/data/www/wikizero.com/index.php Line: 315 Function: require_once A PHP Error was encountered Severity: Warning Message: Invalid argument supplied for foreach() Filename: user/popup_harry_book.php Line Number: 24 Backtrace: File: /var/www/wikizero/data/www/wikizero.com/application/views/user/popup_harry_book.php Line: 24 Function: _error_handler File: /var/www/wikizero/data/www/wikizero.com/application/views/page/index.php Line: 479 Function: view File: /var/www/wikizero/data/www/wikizero.com/application/controllers/Main.php Line: 107 Function: view File: /var/www/wikizero/data/www/wikizero.com/index.php Line: 315 Function: require_once